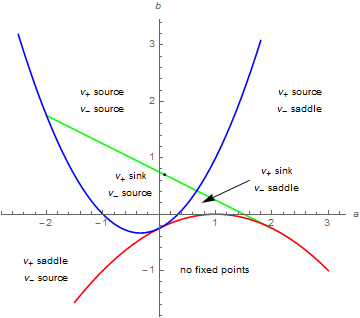
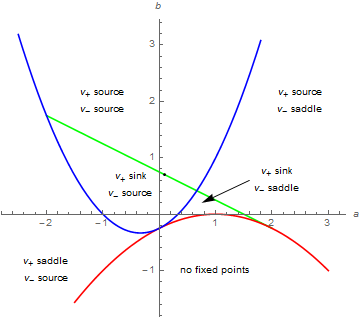
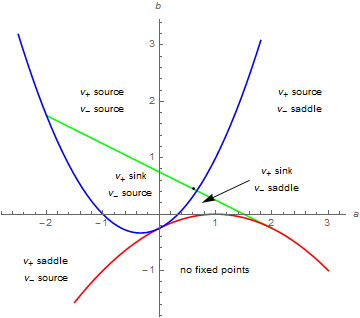
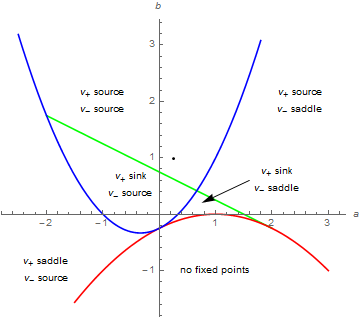
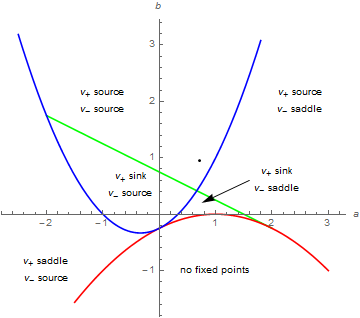
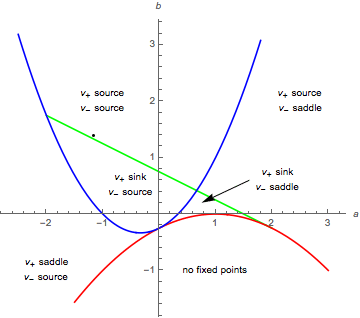
The interesting region in the parameter plane is above the green line, where the eigenvalues of the fixed point v+ are on the unit circle in the complex plane. Above that line, the fixed point is unstable, and there is often a stable limit cycle with quasiperiodic motion.
A few people, for example Scott, showed a transient solution approaching the fixed point, with the eigenvalues just inside the unit circle.


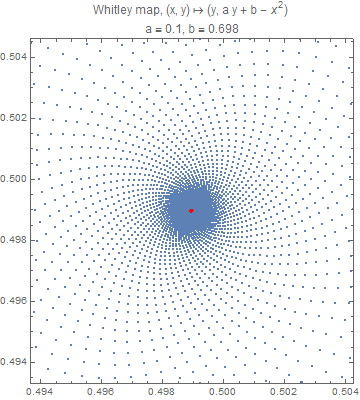
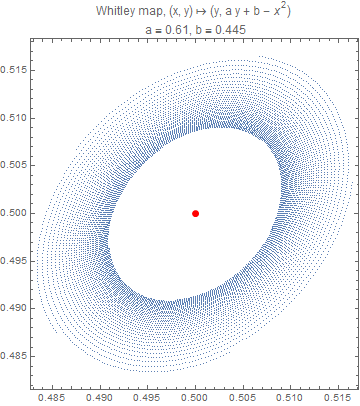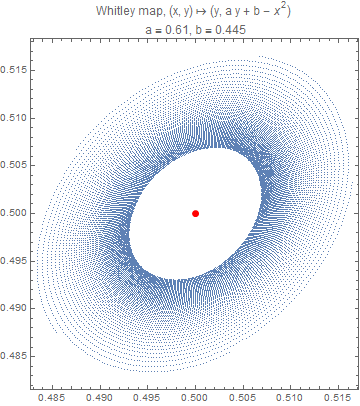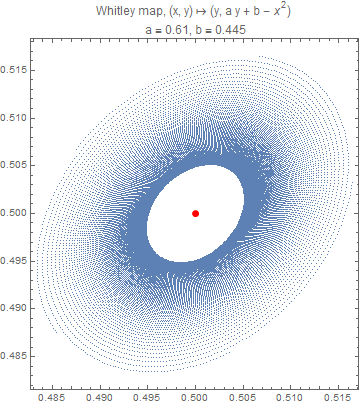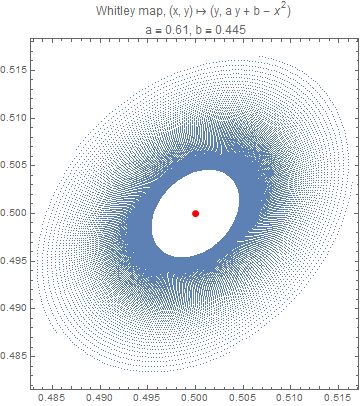
Some people, like Jimie, showed the limit cycle when the parameter is just above the green line. (The eigenvalues are on the unit curcle when a = 0.1 and b = 0.7.)
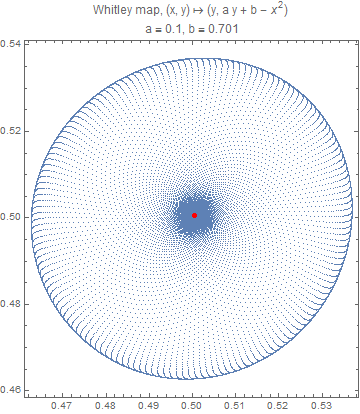
Shehara chose a point on the green line. The animation below shows the orbit with 10, 20, 30, 40, and 50 thousand points. Here the orbit is spiralling
very slowly into the
fixed point whose eigenvalues are on the unit circle.
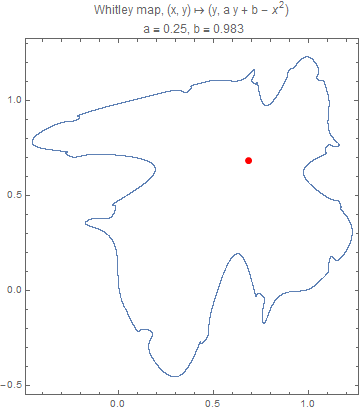
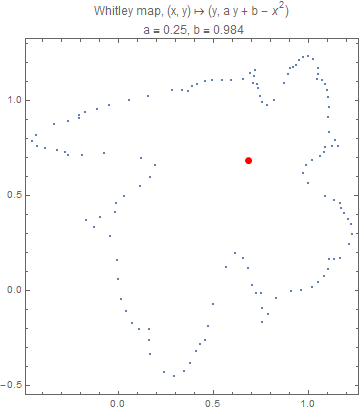
As the parameter point moves up in the a-b plane, the limit cycle grows, and Allie S. submitted the attractor with b = 0.984 in the sequence below, where the limit cycle had broken into a periodic orbit.
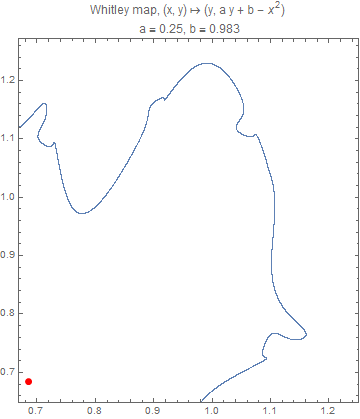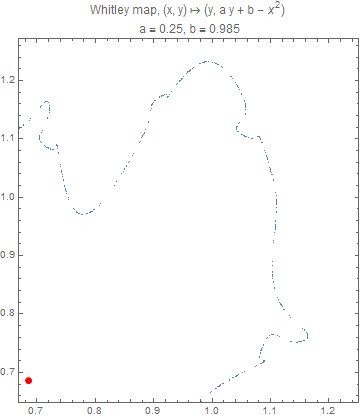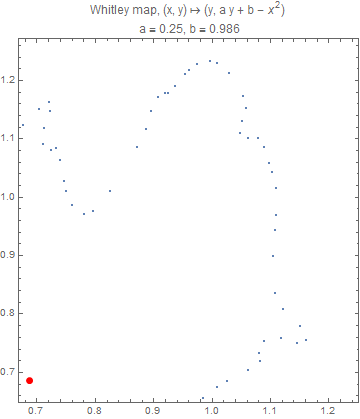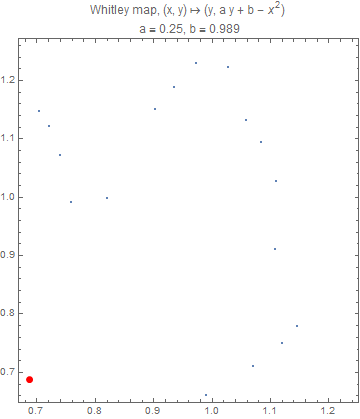
Below is a sequence with several values of b separated by just one or two thousandths. Note how in two attractors, there appear to be gaps in the limit cycle, indicating a ``cantorus''.
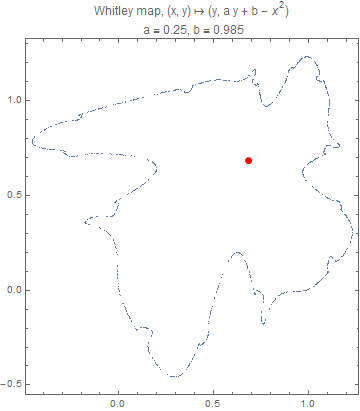
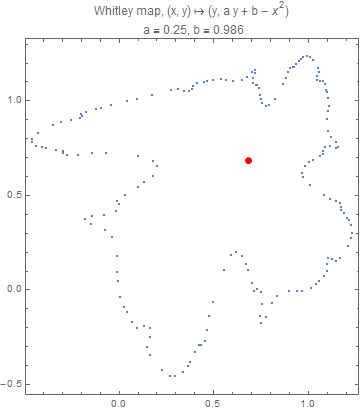
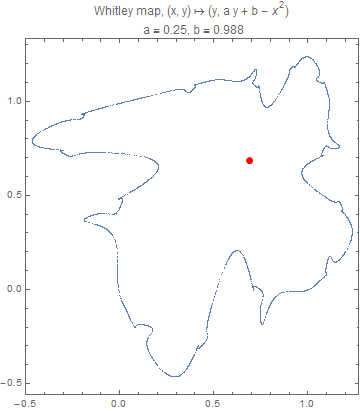
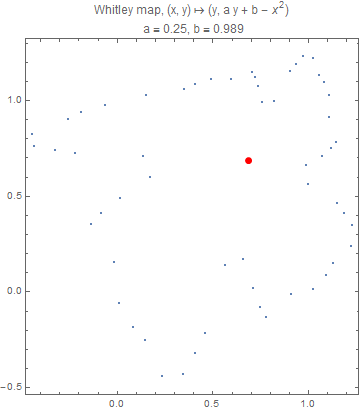
Below is an animation of the upper-right corner of the attractors, for the
same parameter values shown above.
Mandy showed an example of a chaotic transient, where the orbit was actually unbounded. The animation below shows a "crisis" of the attractor: For a = 0.7212 there is an attractor, but
for a = 0.7213 the attractor has gone away and you can see a few dots indicating that the trejectory is unbounded down and to the left.
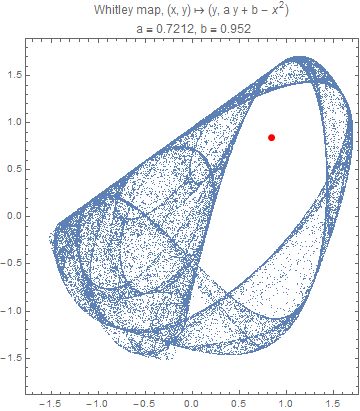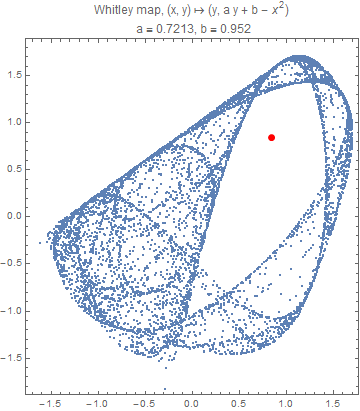
There is another type of crisis, where the attractor does not go away but suddenly changes. Here is an example:
When b = 0.95 the attractor has 5 separate components, and when b = 0.951 there is just one component.
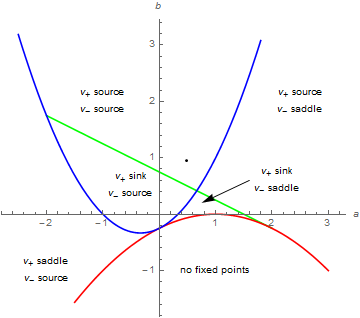
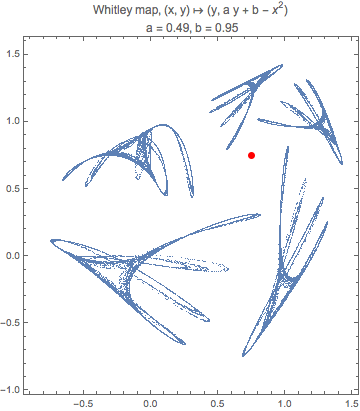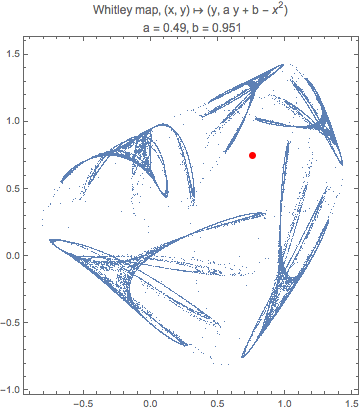
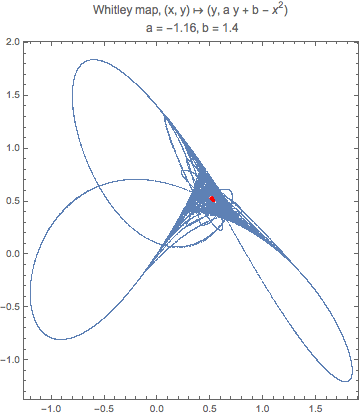
Only one person submitted an attractor for a < 0. At a = -1, b = 1.25, the eigenvalues of v+ are cube roots of unity.
Here is an example of an attractor with some 3-ness near that point.
You can see a page with a selection of Whitley Map attractors from Spring 2012.