Spherical Harmonics

The Spherical Harmonics, Y_ℓ,m(θ, φ), are functions defined on the sphere. They are used to describe the wave function of the electron in a hydrogen atom, oscillations of a soap bubble, etc. The spherical harmonics describe non-symmetric solutions to problems with spherical symmetry.

The Y_ℓ,m’s are complex valued. The radius of the figure is the magnitude, and the color shows the phase, of Y_ℓ,m(θ, φ). These are the numbers on the unit circle: 1 is red, i is purple, -1 is cyan (light blue), and -i is yellow-green.

For each value of ℓ, there are 2ℓ + 1 linearly independent functions Y_ℓ,m, where m = -ℓ, -ℓ+1, ... , ℓ-1, ℓ. I have chosen a different set of 2ℓ + 1 functions, as you see below.

			Y0,0
		Re(Y1,1)	Y1,0	Y1,1
	Re(Y2,2)	Re(Y2,1)	Y2,0	Y2,1	Y2,2
Re(Y3,3)	Re(Y3,2)	Re(Y3,1)	Y3,0	Y3,1	Y3,2	Y3,3

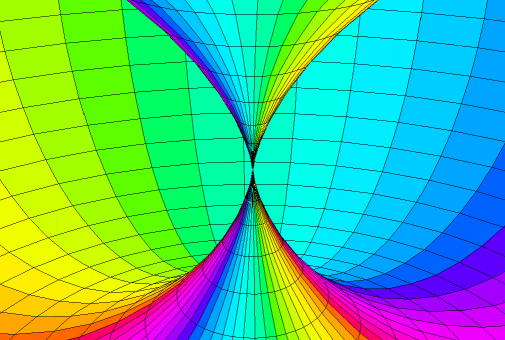
The following figure is called “inside Y_2,2”. My son, Michael, made this by holding down the “Page Up” key until the viewpoint gets inside the surface. (He suggests that you set the figure rotating continuously, and move the viewpoint a bit down before zooming in.)

Oscillations of a Soap Bubble
The volume of the bubble is constant, so Y_0,0 is not used. The center of mass of the bubble is constant, so Y_1,m is not used. The lowest frequency oscillations of a soap bubble are ℓ = 2. The radius of the soap film is r = 1 + ε Y_2,m (θ, φ). The oscillations with different m all have the same frequency. The shape of the oscillations with m = 1 and m = 2 are the same up to a rotation, but the m = 0 oscillation is different.

Physics and Math notation
WARNING: Spherical coordinates are different in physics and mathematics. The symbols θ and φ are switched! The math notation makes r and θ the same in cylincrical and spherical coordinates. DPGraph uses math notation.

These graphs were produced by DPGraph, which is a fast program for viewing 3D objects.