The Central Island

The Central Island

The center of this figure is at (x, y) = (1, 1) = (-1, -1), the corner of the rhombus. Note that this island has more symmetry than the island in Peter Ashwin's figure 2b. The window shown is: 
  0.7375 < xx < 0.7625, 0.99 < y < 1.01,
     where xx = x - a/2 y.
The largest x-y rectangle that fits in the window is 0.99 < x, y < 1.01.

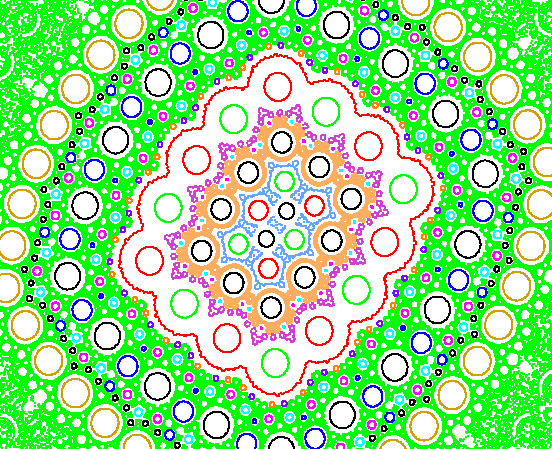
Click on the figure (or here) to explore the Diagonal Symmetries.

The initial conditions of the circular orbits are chosen by the mouse, and were not recorded. The initial conditions of the `chaotic' orbits are: 

Color    i.c.        Comments

green   (-1, .509)   50 million iterates.
red     (-.99991472,0.5061287) Near invariant curve.
magenta (.996, .996) Note the 3-fold pattern
salmon  (-1, .503) 
blue    (1, 1)       i.c. is corner of rhombus.
The green orbit is the same initial condition of the brown orbit in the second blow up. In that figure the brown orbit was only 13 million iterations long, and it stayed confined. Here we see that by 50 million iterates the orbit has `escaped' and it ranges over the whole rhombus. Hence we color it green.
[Back] Overflow map page     [Back] Jim Swift's home page     [Back] Department of Mathematics     [Back] NAU Home Page